.: Gergonne point of a Triangle (remarkable point) :.
Gergonne point of a Triangle (remarkable point):
Dados quaisquer três pontos não colineares A, B e C, o ponto de Gergonne do triângulo ABC
é o ponto Ge que é o encontra das alturas dos vértices até as interseções do incentro com os lados do triângulo.
remarkable point
An remarkable Point of a triangle is the point resulting of the intersection of three lines
that passes through each triangle vertex and its opposite side (cevian lines).
The remarkable fact is the existence of a single point, since there are 3 possible intersections
(one to each pair of lines)!
These lines is named as cevians in honor the mathematician Giovanni Ceva (that published a condition to existence of this single point in 1678).
See: H. S. M. Coxeter, S. L. Greitzer, Geometry Revisited, MAA, 1967.
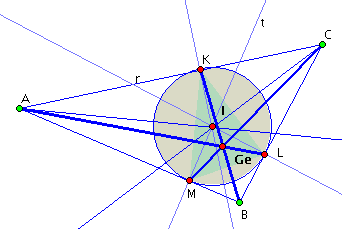
Fig.: static figure representing the Gergonne (note that I is the incenter) point (an special "remarkable point").
Interactive construction with iGeom
Below is presented the Gergone point of a (generic) Triangle in iGeom.
Hands on: try to move the point A (B or C) and observe that:
the segments AL, BK, and CM
has a common point Ge as its intersection (Gergonne point).
In order to move any point (the best) option is: with the "move button"
selected (a single right "click" on it), make a right "click" over
the target point (this means "click" and release the mouse button), then
freely move the mouse around the drawing area.
.